https://doi.org/10.1140/epjc/s10052-024-12449-7
Regular Article - Theoretical Physics
Maximum mass of an anisotropic compact object admitting the modified Chaplygin equation of state in Buchdahl-I metric
Department of Physics, IUCAA Centre for Astronomy Research and Development (ICARD), Cooch Behar Panchanan Barma University, Vivekananda Street, 736101, Cooch Behar, West Bengal, India
Received:
17
October
2023
Accepted:
14
January
2024
Published online:
24
January
2024
In this article, a new class of exact solutions for anisotropic compact objects is presented. Admitting the modified Chaplygin equation of state 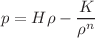 , where H, K and n are constants with
, where H, K and n are constants with 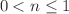 , and employing the Buchdahl-I metric within the framework of the general relativity stellar model is obtained. Recent observations on pulsars and GW events reveal that the observed maximum mass of compact stars detected so far is approximately
, and employing the Buchdahl-I metric within the framework of the general relativity stellar model is obtained. Recent observations on pulsars and GW events reveal that the observed maximum mass of compact stars detected so far is approximately  . Since massive stars cannot be supported by a soft equation of state, a constraint of the equation of state must hold. The choice of a suitable equation of state for the interior matter of compact objects may predict useful information compatible with recent observations. TOV equations have been solved using the modified Chaplygin equation of state to find the maximum mass in this model. In particular, the theory can achieve
. Since massive stars cannot be supported by a soft equation of state, a constraint of the equation of state must hold. The choice of a suitable equation of state for the interior matter of compact objects may predict useful information compatible with recent observations. TOV equations have been solved using the modified Chaplygin equation of state to find the maximum mass in this model. In particular, the theory can achieve 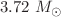 , when
, when  ,
, 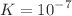 and
and 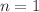 . The model is suitable for describing the mass of pulsars PSR J2215+5135 and PSR J0952-0607 and the mass
. The model is suitable for describing the mass of pulsars PSR J2215+5135 and PSR J0952-0607 and the mass  of the companion star in the GW 190814 event. The
of the companion star in the GW 190814 event. The 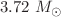 is hardly achievable theoretically in general relativity considering fast rotation effects too. To check the physical viability of this model, we have opted for the stability analysis and energy conditions. We have found that our model satisfies all the necessary criteria to be a physically realistic model.
is hardly achievable theoretically in general relativity considering fast rotation effects too. To check the physical viability of this model, we have opted for the stability analysis and energy conditions. We have found that our model satisfies all the necessary criteria to be a physically realistic model.
© The Author(s) 2024
 Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3. SCOAP3 supports the goals of the International Year of Basic Sciences for Sustainable Development.




