EPJ

2024 Impact factor 4.8
EPJ C - Particles and Fields
Particles and Fields


Eur. Phys. J. C 24, 373-383 (2002)
DOI: 10.1007/s100520200948

Predictions for 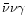${\bar \nu} \nu \gamma$ production at LEP

D. Bardin1, S. Jadach2, 3, 4, T. Riemann2 and Z. Was3

1  Lab. of Nuclear Problems, JINR, 141980 Dubna, Russia
2  Deutsches Elektronen-Synchrotron DESY, 15738 Zeuthen, Germany
3  Institute of Nuclear Physics, ul. Kawiory 26a, 30-055 Cracow, Poland
4  CERN, Theory Division, 1211 Geneva 23, Switzerland

(Received: 30 October 2001 / Revised version: 5 March 2002 / Published online: 22 May 2002 - © Springer-Verlag / Società Italiana di Fisica 2002 )

Abstract
We study predictions for the reaction $e^+e^- \to {\bar \nu} \nu (n \gamma)$. The complete one-loop corrections are taken into account and higher order contributions, in particular those for the observed real photons, are added whenever necessary. The event generator ${\cal KK}$ MC, a general-purpose Monte Carlo generator for the process $e^+e^- \to \bar f f n\gamma$ based on the method of exclusive exponentiation, is used as the environment. We extend its applicability to the process $e^+e^- \to \bar \nu_l \nu_l n\gamma$, $l=e,\mu,\tau$, where the observation of at least a single $\gamma$ is required. The exponentiation is implemented in much the same way as for the s-channel process alone. In particular, all photonic effects present in the case of W exchange, which cannot be included in the s-channel exponentiation scheme, are calculated to a finite order only. The real hard photon matrix element is calculated up to ${\cal O} ({\alpha^2})$. Leading logarithmic contributions of the two-loop corrections and one-loop photonic corrections accompanying real single-photon emission are included. The electroweak corrections are calculated with the DIZET library of the ZFITTER package. Numerical tests and predictions for typical observables are presented.



© Società Italiana di Fisica, Springer-Verlag 2002

Conference announcements