https://doi.org/10.1140/epjc/s10052-024-13461-7
Regular Article
Dynamical system analysis for scalar field potential in teleparallel gravity
1
Department of Mathematics, Birla Institute of Technology and Science-Pilani, Hyderabad Campus, 500078, Hyderabad, India
2
Department of Physics, Indira Gandhi Institute of Technology, Sarang, 759146, Dhenkanal, Odisha, India
d
bivu@hyderabad.bits-pilani.ac.in
Received:
20
July
2024
Accepted:
6
October
2024
Published online:
21
October
2024
In this paper, we have presented a power law cosmological model and its dynamical system analysis in 
gravity, where T is the torsion scalar and 
is the canonical scalar field. The two well-motivated forms of the non-minimal coupling function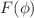 
, the exponential form and the power law form, with exponential scalar field potential, are investigated. The dynamical system analysis is performed by establishing the dimensionless dynamical variables, and the critical points were obtained. The evolution of standard density parameters is analysed for each case. The behaviour of the equation of state (EoS) and deceleration parameter agree with the result of recent cosmological observations. The model parameters are constrained using the existence and the stability conditions of the critical points describing different epochs of the evolution of the Universe.
© The Author(s) 2024
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3.
