https://doi.org/10.1140/epjc/s10052-024-13372-7
Regular Article - Theoretical Physics
Classical mechanics in noncommutative spaces: confinement and more
1
Centro de Matemática, Computação e Cognição, Universidade Federal do ABC, Santo André, SP, Brazil
2
Dipartimento di Fisica “E. Pancini”, Università di Napoli Federico II, Complesso Universitario di Monte S. Angelo Edificio 6, 80126, via Cintia, Naples, Italy
3
Physics Faculty, Tomsk State University, Lenin Ave. 36, 634050, Tomsk, Russia
4
INFN-Sezione di Napoli, Complesso Universitario di Monte S. Angelo Edificio 6, via Cintia, 80126, Napoli, Italy
a
vladislav.kupriyanov@gmail.com
Received:
3
August
2024
Accepted:
13
September
2024
Published online:
16
October
2024
We consider a semi-classical approximation to the dynamics of a point particle in a noncommutative space. In this approximation, the noncommutativity of space coordinates is described by a Poisson bracket. For linear Poisson brackets, the corresponding phase space is given by the cotangent bundle of a Lie group, with the Lie group playing the role of a curved momentum space. We show that the curvature of the momentum space may lead to rather unexpected physical phenomena such as an upper bound on the velocity of a free nonrelativistic particle, bounded motion for repulsive central force, and no-fall-into-the-centre for attractive Coulomb potential. We also consider a superintegrable Hamiltonian for the Kepler problem in 3-space with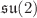 
noncommutativity. The leading correction to the equations of motion due to noncommutativity is shown to be described by an effective monopole potential.
© The Author(s) 2024
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3.
