https://doi.org/10.1140/epjc/s10052-024-13272-w
Regular Article - Theoretical Physics
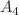 modular invariance and the strong CP problem
modular invariance and the strong CP problem
1
INFN/SISSA, Via Bonomea 265, 34136, Trieste, Italy
2
Kavli IPMU (WPI), UTIAS, The University of Tokyo, 277-8583, Kashiwa, Chiba, Japan
3
Department of Physics, Niigata University, Ikarashi 2, 950-2181, Niigata, Japan
4
Institute of Nuclear Research and Nuclear Energy, Bulgarian Academy of Sciences, 1784, Sofia, Bulgaria
b
morimitsutanimoto@yahoo.co.jp
Received:
1
July
2024
Accepted:
19
August
2024
Published online:
10
September
2024
We present simple effective theory of quark masses, mixing and CP violation with level 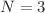 (
(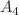 ) modular symmetry, which provides solution to the strong CP problem without the need for an axion. The vanishing of the strong CP-violating phase
) modular symmetry, which provides solution to the strong CP problem without the need for an axion. The vanishing of the strong CP-violating phase 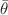 is ensured by assuming CP to be a fundamental symmetry of the Lagrangian of the theory. The CP symmetry is broken spontaneously by the vacuum expectation value (VEV) of the modulus
is ensured by assuming CP to be a fundamental symmetry of the Lagrangian of the theory. The CP symmetry is broken spontaneously by the vacuum expectation value (VEV) of the modulus  . This provides the requisite large value of the CKM CP-violating phase while the strong CP phase
. This provides the requisite large value of the CKM CP-violating phase while the strong CP phase 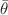 remains zero or is tiny. Within the considered framework we discuss phenomenologically viable quark mass matrices with three types of texture zeros, which are realized by assigning both the left-handed and right-handed quark fields to
remains zero or is tiny. Within the considered framework we discuss phenomenologically viable quark mass matrices with three types of texture zeros, which are realized by assigning both the left-handed and right-handed quark fields to 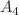 singlets
singlets 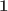 ,
, 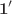 and
and 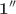 with appropriate weights. The VEV of
with appropriate weights. The VEV of  is restricted to reproduce the observed CKM parameters. We discuss cases in which the modulus VEV is close to the fixed points i,
is restricted to reproduce the observed CKM parameters. We discuss cases in which the modulus VEV is close to the fixed points i,  and
and  . In particular, we focus on the VEV of
. In particular, we focus on the VEV of  , which gives the absolute minima of the supergravity-motivated modular- and CP-invariant potentials for the modulus
, which gives the absolute minima of the supergravity-motivated modular- and CP-invariant potentials for the modulus  , so called, modulus stabilisation. We present a successful model, which is consistent with the modulus stabilisation close to
, so called, modulus stabilisation. We present a successful model, which is consistent with the modulus stabilisation close to  .
.
© The Author(s) 2024
 Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3.




