https://doi.org/10.1140/epjc/s10052-023-12136-z
Regular Article - Theoretical Physics
Geodesic deviation equation in generalized hybrid metric-Palatini gravity
Department of Physics, Azarbaijan Shahid Madani University, 53714-161, Tabriz, Iran
Received:
6
July
2023
Accepted:
12
October
2023
Published online:
11
November
2023
In the context of general relativity, the geodesic deviation equation (GDE) relates the Riemann curvature tensor to the relative acceleration of two neighboring geodesics. In this paper, we consider the GDE for the generalized hybrid metric-Palatini gravity and apply it in this model to investigate the structure of time-like, space-like, and null geodesics in the homogeneous and isotropic universe. We propose a particular case 
to study the numerical behavior of the deviation vector 
and the observer area–distance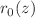 
with respect to redshift z. Also, we consider the GDE in the framework of the scalar–tensor representation of the generalized hybrid metric-Palatini gravity, i.e., 
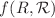, in which the model can be considered as dynamically equivalent to a gravitational theory with two scalar fields. Finally, we extend our calculations to obtain the modification of the Mattig relation in this model.
© The Author(s) 2023
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3. SCOAP3 supports the goals of the International Year of Basic Sciences for Sustainable Development.
