https://doi.org/10.1140/epjc/s10052-021-09542-6
Regular Article - Theoretical Physics
Reconstructing inflation in scalar-torsion 
gravity
Instituto de Física, Pontificia Universidad Católica de Valparaíso, Casilla, 4950, Valparaíso, Chile
Received:
10
June
2021
Accepted:
8
August
2021
Published online:
14
August
2021
It is investigated the reconstruction during the slow-roll inflation in the most general class of scalar-torsion theories whose Lagrangian density is an arbitrary function of the torsion scalar T of teleparallel gravity and the inflaton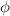 
. For the class of theories with Lagrangian density 
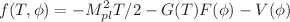, with 
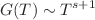and the power s as constant, we consider a reconstruction scheme for determining both the non-minimal coupling function 
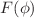and the scalar potential 
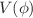through the parametrization (or attractor) of the scalar spectral index 
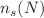and the tensor-to-scalar ratio r(N) as functions of the number of 
folds N. As specific examples, we analyze the attractors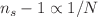 
and 
, as well as the case 
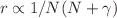with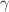 
a dimensionless constant. In this sense and depending on the attractors considered, we obtain different expressions for the function 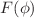and the potential 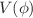, as also the constraints on the parameters present in our model and its reconstruction.
© The Author(s) 2021
Open Access This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons licence, and indicate if changes were made. The images or other third party material in this article are included in the article’s Creative Commons licence, unless indicated otherwise in a credit line to the material. If material is not included in the article’s Creative Commons licence and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly from the copyright holder. To view a copy of this licence, visit http://creativecommons.org/licenses/by/4.0/.
Funded by SCOAP3
