https://doi.org/10.1140/epjc/s10052-020-8108-2
Regular Article - Theoretical Physics
Cyclicity of all anti-NMHV and 
tree amplitudes in 
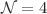SYM
Max Planck Institute for Gravitational Physics (Albert Einstein Institute), 14476, Potsdam, Germany
* e-mail: jrao@aei.mpg.de
Received:
25
April
2020
Accepted:
1
June
2020
Published online:
16
June
2020
This article proves the cyclicity of anti-NMHV and tree amplitudes in planar 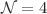SYM up to any number of external particles as an interesting application of positive Grassmannian geometry. In this proof the two-fold simplex-like structures of tree amplitudes introduced in 1609.08627 play a key role, as the cyclicity of amplitudes will induce similar simplex-like structures for the boundary generators of homological identities. For this purpose, we only need a part of all distinct boundary generators, and the relevant identities only involve BCFW-like cells. The manifest cyclic invariance in this geometric representation reflects one of the invariant characteristics of amplitudes, though they are obtained by the scheme-dependent BCFW recursion relation.
© The Author(s), 2020
