https://doi.org/10.1140/epjc/s10052-018-5966-y
Regular Article - Theoretical Physics
Smoothed one-core and core–multi-shell regular black holes
Engineering Faculty, Başkent University, Bağlıca Campus, 06810, Ankara, Turkey
* e-mail: azreg@baskent.edu.tr
Received:
1
November
2017
Accepted:
2
June
2018
Published online:
9
June
2018
We discuss the generic properties of a general, smoothly varying, spherically symmetric mass distribution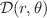 
, with no cosmological term (
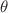is a length scale parameter). Observing these constraints, we show that (1.) the de Sitter behavior of spacetime at the origin is generic and depends only on 
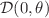, (2.) the geometry may posses up to 
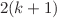horizons depending solely on the total mass M if the cumulative distribution of 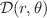has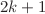 
inflection points, and (3.) no scalar invariant nor a thermodynamic entity diverges. We define new two-parameter mathematical distributions mimicking Gaussian and step-like functions and reduce to the Dirac distribution in the limit of vanishing parameter 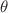. We use these distributions to derive in closed forms asymptotically flat, spherically symmetric, solutions that describe and model a variety of physical and geometric entities ranging from noncommutative black holes, quantum-corrected black holes to stars and dark matter halos for various scaling values of 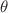. We show that the mass-to-radius ratio 
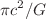is an upper limit for regular-black-hole formation. Core–multi-shell and multi-shell regular black holes are also derived.
© The Author(s), 2018
