Eur. Phys. J. C (2018) 78: 412
https://doi.org/10.1140/epjc/s10052-018-5850-9

Regular Article - Theoretical Physics

The quantum cat map on the modular discretization of extremal black hole horizons

¹ NCSR “Demokritos”, Institute of Nuclear and Particle Physics, 15310, Aghia Paraskevi, Attiki, Greece
² Physics Department, University of Athens, Zografou University Campus, 15771, Athens, Greece
³ CNRS-Institut Denis Poisson (UMR 7013), Université de Tours, Université d’Orléans, Parc Grandmont, 37200, Tours, France

^* e-mail: stam.nicolis@lmpt.univ-tours.fr

Received: 25 October 2017
Accepted: 26 April 2018
Published online: 24 May 2018

Abstract

Based on our recent work on the discretization of the radial 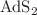geometry of extremal BH horizons, we present a toy model for the chaotic unitary evolution of infalling single-particle wave packets. We construct explicitly the eigenstates and eigenvalues for the single-particle dynamics for an observer falling into the BH horizon, with as time evolution operator the quantum Arnol’d cat map (QACM). Using these results we investigate the validity of the eigenstate thermalization hypothesis (ETH), as well as that of the fast scrambling time bound (STB). We find that the QACM, while possessing a linear spectrum, has eigenstates, which are random and satisfy the assumptions of the ETH. We also find that the thermalization of infalling wave packets in this particular model is exponentially fast, thereby saturating the STB, under the constraint that the finite dimension of the single-particle Hilbert space takes values in the set of Fibonacci integers.