EPJ

2024 Impact factor 4.8
EPJ C - Particles and Fields
Particles and Fields
Eur. Phys. J. C 7, 571-593
DOI 10.1007/s100529901061

Measurement of the strong coupling constant 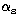\ensuremath{\alpha_{\rm s}} and the vector and axial-vector spectral functions in hadronic tau decays

The OPAL Collaboration

K. Ackerstaff8 - et al.

8CERN, European Organisation for Particle Physics, CH-1211 Geneva 23, Switzerland

Received: 23 June 1998 / Published online: 19 February 1999

Abstract
The spectral functions of the vector current and the axial-vector current have been measured in hadronic $\tau$ decays using the OPAL detector at LEP. Within the framework of the Operator Product Expansion a simultaneous determination of the strong coupling constant \ensuremath{\alpha_{\rm s}}, the non-perturbative operators of dimension 6 and 8 and of the gluon condensate has been performed. Different perturbative descriptions have been compared to the data. The Contour Improved Fixed Order Perturbation Theory gives $\ensuremath{\alpha_{\rm s}}(m_\tau^2) = 0.348 \pm \ensuremath{ 0.009}_{\rm exp} \pm \ensuremath{ 0.019}_{\rm theo}$ at the $\tau$-mass scale and $\ensuremath{\alpha_{\rm s}}(\ensuremath{m_\mathrm{Z}^2}) = \ensuremath{ 0.1219}\pm \ensuremath{ 0.0010}_{\rm exp} \pm \ensuremath{ 0.0017}_{\rm theo}$ at the Z0-mass scale. The values obtained for $\ensuremath{\alpha_{\rm s}}(\ensuremath{m_\mathrm{Z}^2})$ using Fixed Order Perturbation Theory or Renormalon Chain Resummation are $2.3\,\%$ and $4.1\,\%$ smaller, respectively. The running of the strong coupling between $s_0 \simeq 1.3\,{\rm GeV}^2$ and $s_0 = m_\tau^2$ has been tested from direct fits to the integrated differential hadronic decay rate $R_\tau(s_0)$.A test of the saturation of QCD sum rules at the $\tau$-mass scale has been performed.

Copyright Springer-Verlag

Conference announcements